Prologue
Embark on an intellectual journey into the captivating realm of arithmetic sequences, where the unraveling of a1 holds the key to comprehending their intricate progression. This comprehensive guide will equip you with the necessary tools to master this mathematical enigma, demystifying its secrets and empowering you to wield its power.
Delving into Arithmetic Sequences
Arithmetic sequences, a cornerstone of mathematical exploration, are captivating for their consistency and elegance. They represent a series of numbers that increase or decrease by a constant difference known as the common difference (d). The first term in the sequence is often denoted by a1, setting the stage for the sequential dance that follows. Understanding a1 is akin to unraveling the blueprint of the entire sequence, providing invaluable insights into its behavior and applications.
Unveiling a1: The Mathematical Key
The key to unlocking a1 lies within the formula:
a1 = a – (n-1)d
Where:
– a1 is the first term
– a is any term in the sequence
– n is the position of the term “a” in the sequence
– d is the common difference
This formula provides a direct path to determining a1, enabling you to pinpoint the genesis of any arithmetic sequence. By skillfully manipulating this equation, you can decipher the hidden pattern and gain control over the progression.
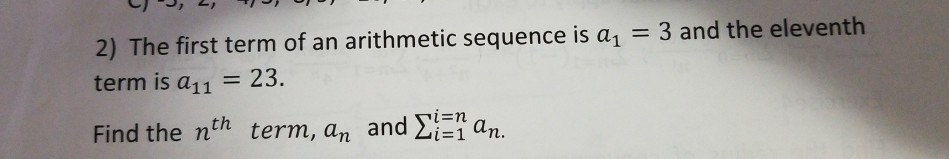
Image: www.chegg.com
Navigating Sample Problems
Let’s illuminate the process with an illuminating example: Given an arithmetic sequence with an 8th term of 24 and a common difference of 3, what is the first term, a1?
Employing the formula, we have:
24 = a1 + (8-1)3
Solving for a1, we arrive at:
24 = a1 + 21
Subtracting 21 from both sides, we uncover:
a1 = 3
Armed with this newfound knowledge, we can confidently assert that the first term of the sequence is indeed 3. This empowers us to reconstruct the entire sequence, term by term, unraveling its hidden progression.
Applications of Arithmetic Sequences
Beyond theoretical exploration, arithmetic sequences possess practical applications that span a diverse array of fields:
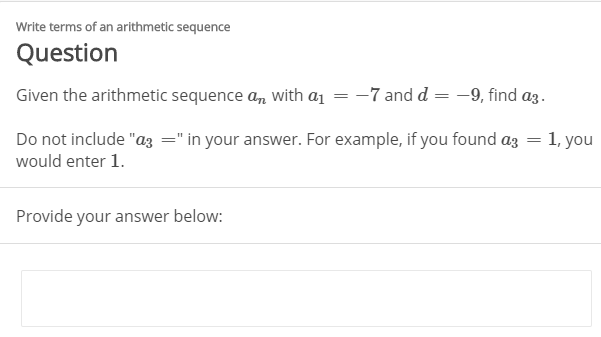
Image: www.bartleby.com
How To Find A1 In Arithmetic Sequence
Conclusion
The mastery of a1 in arithmetic sequences grants you the power to unravel the hidden patterns that govern this captivating mathematical construct. With the formula and problem-solving techniques presented in this comprehensive guide, you are now equipped to tackle any challenge that comes your way. Remember, the pursuit of knowledge is an ongoing journey, and the exploration of arithmetic sequences is an exciting chapter in that journey. May this guide serve as your constant companion, illuminating your path to mathematical enlightenment.